MATHEMATICS 2009
Comptt.
Set III - Delhi
Comptt.
Set III - Delhi
Time allowed : 3 hours
Maximum marks : 80
Maximum marks : 80
General Instructions :
1. All questions are compulsory.
2. The Question Paper consists of 30 questions divided into 4 Sections - A, B, C and D. Section A comprises of ten questions of 01 mark each, Section B comprises of five questions of 02 marks each, Section C comprises of ten questions of 03 marks each and Section D comprises of five questions of 06 marks each.
3. All questions in Section A are to be answered in one word, one sentence or as per the exact requirement of the question.
4. There is no overall choice. However, an internal choice has been provided in one question of 02 marks each, three questions of 03 marks each and two questions of 06 marks each. You have to attempt only one of the alternatives in all such questions.
5. In question on construction, the drawings should be neat and exactly as per the given measurements.
6. Use of calculators is not permitted. However, you may ask for mathematical tables.
1. All questions are compulsory.
2. The Question Paper consists of 30 questions divided into 4 Sections - A, B, C and D. Section A comprises of ten questions of 01 mark each, Section B comprises of five questions of 02 marks each, Section C comprises of ten questions of 03 marks each and Section D comprises of five questions of 06 marks each.
3. All questions in Section A are to be answered in one word, one sentence or as per the exact requirement of the question.
4. There is no overall choice. However, an internal choice has been provided in one question of 02 marks each, three questions of 03 marks each and two questions of 06 marks each. You have to attempt only one of the alternatives in all such questions.
5. In question on construction, the drawings should be neat and exactly as per the given measurements.
6. Use of calculators is not permitted. However, you may ask for mathematical tables.
SECTION A
Q. 1. After how many decimal places, will the decimal expansion of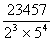 
terminate?Ans . Four (4) decimal places.
Sol .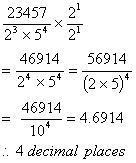
Q. 2. If the product of zeroes of the quadratic polynomial p(x) = (k – 2)x2 – 4x + k, is 3, write the value of k.
Ans. k = 3
Sol .
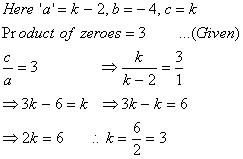
Q. 3. For what value of ‘a’ does the following pair of linear equations have infinitely many solutions?
4x – 3y – (a – 2) = 0 8x – 6y – a = 0.
Ans. a = 4
Sol.
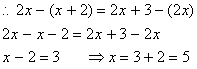
Q. 5. Write the value of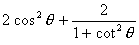 
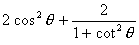
Ans . 2
Sol .
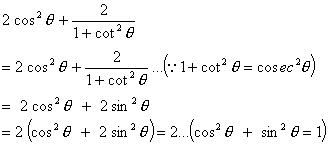
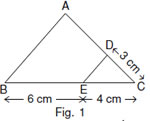
Q. 6. In Fig. 1, DE || AB, find the length of AC.
Ans. 7.5
Sol .
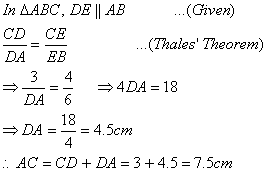
Q. 7. In Fig. 2, PA and PB are tangents drawn from a point P to the circle with centre O. If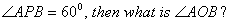 
Ans . 120
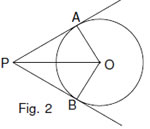
Sol .
Q. 8. What is the angle subtended at the centreof a circle of radius 7 cm, by an arc of length 11 cm?
Ans . 90°
Sol .
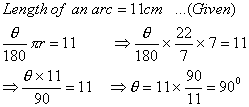
Q. 9. A box contains 3 blue, 2 white and 4 red marbles. If a marble is drawn at random from the box, what is the probability that it will not be a whitemarble? 1
Sol. Total no. of marbles = 3 + 2 + 4 = 9No. of non-white marbles = 3 + 4 = 7
P(not a white marble) =
Q. 10. Determine k so that 3k – 2, 4k – 6 and k + 2 are three consecutive terms of an A.P. 1
Sol. 3k – 2, 4k – 6 and k + 2 are three consecutive
terms of an A.P.
4k – 6 – (3k – 2) = k + 2 – (4k – 6)
…[If a, b, c are in A.P. then b – a = c – b]
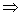4k – 6 – 3k + 2 = k + 2 – 4k + 6
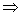k – 4 = – 3k + 8 k + 3k = 8 + 4
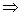4k = 12 
k = 3
terms of an A.P.
4k – 6 – (3k – 2) = k + 2 – (4k – 6)
…[If a, b, c are in A.P. then b – a = c – b]
4k – 6 – 3k + 2 = k + 2 – 4k + 6 k – 4 = – 3k + 8 k + 3k = 8 + 4 4k = 12 k = 3SECTION B
Questions number 11 to 15 carry 2 marks each.
Q. 11. On dividing x3 – 3x2 + x + 2 by a polynomial g(x), the quotient and remainder were x – 2 and – 2x + 4, respectively. Find g(x).
Sol . Dividend = Divisor X Quotient + Remainder
Q. 12. If tan (2A) = cot(A – 21°), where 2A is an acute angle, then find the value of A.
Sol :
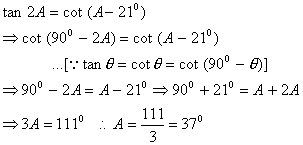
Q. 13. If A(–2, 4), B(0, 0) and C(4, 2) are the vertices of a Δ ABC, then find the length of median through the vertexA.
Ans.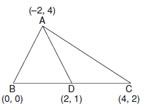 
Sol .
Q. 14. If sec 2A = cosec (A – 27°), then find the value of A, where 2A is an acute angle. 2
Sol . sec 2A = cosec(A – 27°)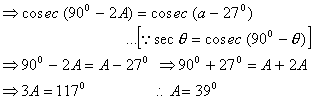
Q. 15. Find the point on y-axis which is equidistant from (– 5, 2) and (9, – 2). 2
Sol. Let P(0, y) be any point on y-axis
Let A(– 5, 2), B(9, – 2)
PA = PB …(Given)
PA2 = PB2 …(Squaring both sides)
Let A(– 5, 2), B(9, – 2)
PA = PB …(Given)
PA2 = PB2 …(Squaring both sides)
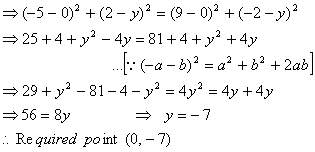
Q. 15. Find the probability that a number selected at random from the numbers 3, 4, 5,…, 25 is prime.
Sol . Total given numbers = 23 Prime numbers are 3, 5, 7, 11, 13, 17, 19, 23 = 8
Prob. (Prime number) =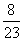
Prob. (Prime number) =
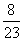
SECTION C
Questions number 16 to 25 carry 3 marks each.
Q. 16. Prove that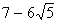 
is an irrational number.
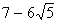is an irrational number.Sol.
Let us assume, to the contrary, that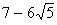
is rational That is, we can find coprimes a and b 
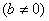such that
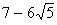is rational That is, we can find coprimes a and b 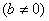such that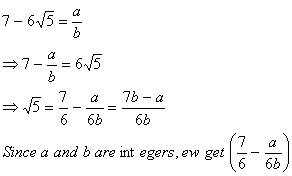 
is rational, and so
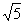is rational But this contradicts the fact that 
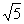is irrational. This contradiction has arisen because of our incorrect assumption that 
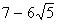is rational. So, we conclude that 
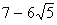is irrational.Q. 17. Check graphically whether the pair of equations 3x + 5y = 15 and x – y = 5 is consistent. Also find the coordinates of the points where the graphs of the equations meet the y-axis.
Sol .
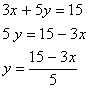
| x | 0 | -5 | 5 |
| y | 3 | 6 | 0 |
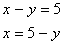
| x | 5 | 7 | 3 |
| y | 0 | 2 | -2 |
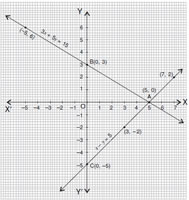
By plotting the points and joining them, the lines intersect at A(5, 0)
x = 5, y = 0
The pair of given equation is consistent.
The equation 3x + 5y = 15 meets the y-axis at
B(0, 3)The equation x – y = 5 meets the y-axis at C(0, – 5)
x = 5, y = 0The pair of given equation is consistent.The equation 3x + 5y = 15 meets the y-axis at
B(0, 3)The equation x – y = 5 meets the y-axis at C(0, – 5)
Or
Places A and B are 160 km apart on a highway. One car starts from A and another from B at the
same time. If the cars travel in the same direction at different speeds, they meet in 8 hours, but if
they travel towards each other, they meet in 2 hours. What are the speeds of the two cars?
same time. If the cars travel in the same direction at different speeds, they meet in 8 hours, but if
they travel towards each other, they meet in 2 hours. What are the speeds of the two cars?
Sol .
Let the speed of 1st car starting from place A = x km/hr
and the speed of 2nd car starting from place B = y km/hr
When two cars travel in the same direction
Distance covered by 1st car in 8 hours
= 160 km + Distance covered by 2nd car
8x = 8y + 160 …( Q Distance = Speed
time)
x = y + 20 …(i) (Dividing both sides by 8)
When two cars travel towards each other
2x + 2y = 160 …(
D = S
T)
x + y = 80 …(Dividing both sides by 2)
y + 20 + y = 80 …[From (i)]
2y = 80 – 20 = 60
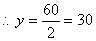
From (i), x = 30 + 20 = 50
Speed of car starting from place A = 50 km/hr
and speed of car starting from place B = 30 km/hr.
and the speed of 2nd car starting from place B = y km/hr
When two cars travel in the same direction
Distance covered by 1st car in 8 hours
= 160 km + Distance covered by 2nd car
8x = 8y + 160 …( Q Distance = Speed
time)x = y + 20 …(i) (Dividing both sides by 8)
When two cars travel towards each other
2x + 2y = 160 …(
D = ST)x + y = 80 …(Dividing both sides by 2)
y + 20 + y = 80 …[From (i)]
2y = 80 – 20 = 60
From (i), x = 30 + 20 = 50
Speed of car starting from place A = 50 km/hrand speed of car starting from place B = 30 km/hr.
Q. 18. Find the roots of the equation :
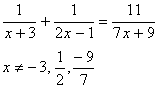
Sol .
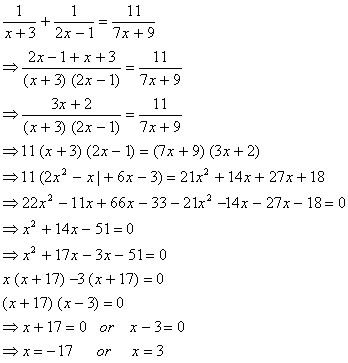
Q. 19. Find the sum of all three digit numbers which are divisible by 7.
Sol. To find : 105 + 112 + 119 + … + 994
Ist term, a = 105
Common difference, d = 112 – 105 = 7
an = 994
a + (n – 1)d = 994
105 + (n – 1)7 = 994
(n – 1)7 = 994 – 105 = 889
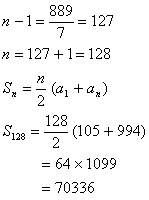
Ist term, a = 105
Common difference, d = 112 – 105 = 7
an = 994
a + (n – 1)d = 994
105 + (n – 1)7 = 994
(n – 1)7 = 994 – 105 = 889
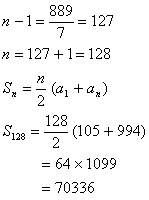
Q. 20. Prove that :
Or
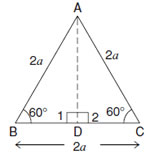
Using Geometry, find the value of sin 60°.
Sol.
Δ ABC be an equilateral, Let each side be 2a. Since each angle in equilateral Δ is 60°
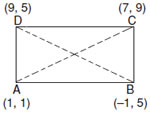
Q. 21. Show that the points (1, 1), (–1, 5), (7, 9) and (9, 5) taken in that order, are the vertices of a rectangle.
Sol. Let A(1, 1), B(– 1, 5), C(7, 9) and D(9, 5) Using Distance formula
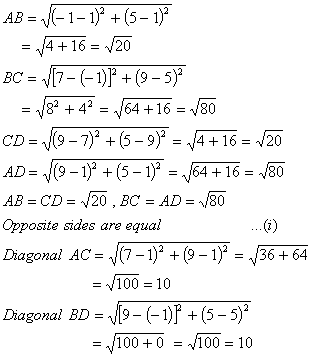
Diagonal AC = Daigonal BD = 10
∴ Diagonals are equal …(ii)
From (i) and (ii), we get
Points A, B, C and D are the vertices of a rectangle.
∴ Diagonals are equal …(ii)
From (i) and (ii), we get
Points A, B, C and D are the vertices of a rectangle.
Q. 22. Find the area of the triangle formed by joining the mid-points of the sides of the trianglewhose vertices are (2, 2), (4, 4) and (2, 6).
Sol. Let A(2, 2), B(4, 4), C(2, 6)
Mid-point of AB,
Mid-point of AB,
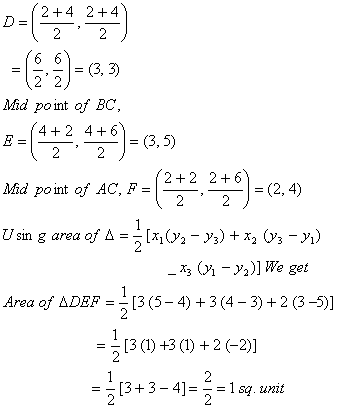
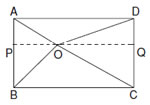
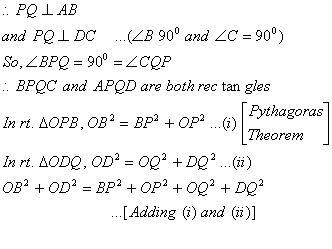
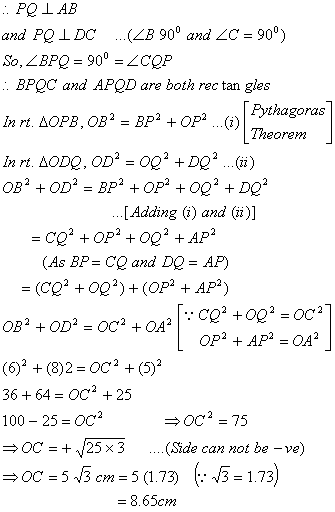
Q. 23. In Fig. 4, O is any point inside a rectangle ABCD such that OB = 6 cm, OD = 8cm and OA = 5 cm.Find the length of OC.
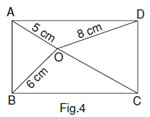
Sol. Through O, draw
PQ || BC
So that P lies on AB
and Q lies on DC.
PQ || BC
PQ || BC
So that P lies on AB
and Q lies on DC.
PQ || BC
Q. 24. Find the roots of the equation .
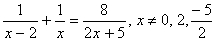
Sol .
Q. 25. Find the sum of all three digit numbers which are divisible by 11. 3
Sol. To find : 110 + 121 + 132 + … + 990
1st term, a = 110
1st term, a = 110
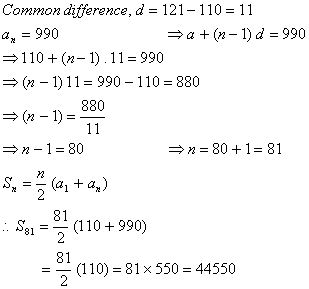
SECTION D
Questions number 26 to 30 carry 6 marks each.
Q. 26. The diagonal of a rectangular field is 60 metres more than the shorter side. If the longer side is 30 metres more than the shorter side, find the sides of the field.
Sol. Let shorter side, AB = x m then Diagonal, AC = (x + 60) m and longer side,
BC = (x + 30) m In rt. Δ ABC,
BC = (x + 30) m In rt. Δ ABC,
Q. 27. A boy, who’s eye level is 1.3 m from the ground, spots a balloon moving with the wind in a horizontal line at some height from the ground. The angle of elevation of the balloon from the eyes of the boy at any instant is 60°. After 2 seconds, the angle of elevation reduces to 30°. If the speed of wind at that moment is 29 3 m/s, then find the height of the balloon from the ground.
Sol. Let AP be the boy, C and E are two positions
of the balloon. Speed of wind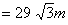
Distance travelled by the balloon in 2 seconds,
CE = BD =
Let AB = x m and BC = DE = y m
of the balloon. Speed of wind
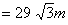
Distance travelled by the balloon in 2 seconds,
CE = BD =
Let AB = x m and BC = DE = y m
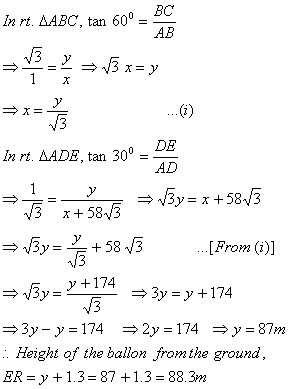
Or
A statue, 1.5 m tall, stands on the top of a pedestal. From a point on the ground, the angle of elevation of the top of the statue is 45° and from the same point the angle of elevation of the top of the pedestal is 30°. Find the height of the pedestal.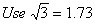 
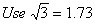
Sol. Let BC = h m
be the pedestal.
CD be the statue.
Distance from the
point be AB = x m
In rt.,
be the pedestal.
CD be the statue.
Distance from the
point be AB = x m
In rt.,
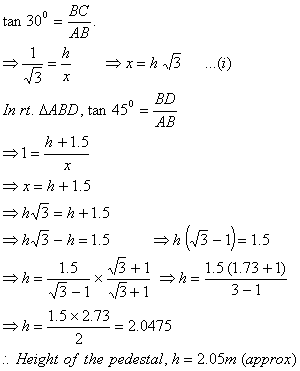
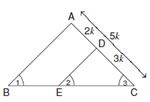
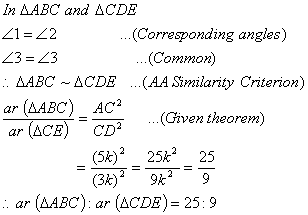
Q. 28 . Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides. Using the above, do the following .
If D is a point on the side AC of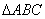 
such that
AD : DC = 2 : 3, and E is a point on BC such that
DE || AB; then find the ratio of areas of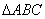
and 
.
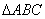such thatAD : DC = 2 : 3, and E is a point on BC such that
DE || AB; then find the ratio of areas of
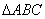and .Sol. Part I: See Theorem 2,
Page (xxxi).
Part II:
Let AD = 2k, CD = 3k
Then AC = 2k + 3k = 5k
Page (xxxi).
Part II:
Let AD = 2k, CD = 3k
Then AC = 2k + 3k = 5k
Q. 29. The table below shows the daily expenditure on food of 30 households in a locality.
| Daily Expenditure (in Rs) | Number of households |
| 100 – 150 | 6 |
| 150 – 200 | 7 |
| 200 – 250 | 12 |
| 250 – 300 | 3 |
| 300 – 350 | 2 |
Find the mean and median daily expenditure on food. 6
Sol .
| Daily Expenditure (in Rs) | Number of fi | xi | 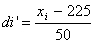 | Fidi’ | c.f | |
| 100 – 150 | 6 | 125 | -2 | -12 -7 | 16 | 6 |
| 150 – 200 | 7 | 175 | -1 | 13 | ||
| 200 – 250 | 12 | 225 | 0 | 0 | 25 | |
| 250 – 300 | 3 | 275 | 1 | 3 4 | 7 | 28 |
| 300 – 350 | 2 | 325 | 2 | 30 | ||
 |  | |||||
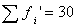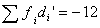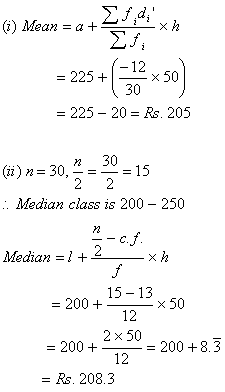
Q. 30. A train travels 288 km at a uniform speed. If the speed had been 4 km/hr more, it would have taken 1 hour less for the same journey. Find the speed of the train. 6
Sol.
Let the normal speed of the train
= x km/hr
Then the increased speed of the train
= (x + 4) km/hr
Distance = 288 km
= x km/hr
Then the increased speed of the train
= (x + 4) km/hr
Distance = 288 km
According to the question
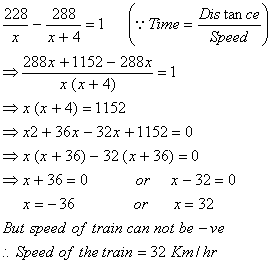
No comments:
Post a Comment